What four quantities are needed to accurately describe a gas?
5.2: Gas Pressure and Its Measurement
- Page ID
- 83769
Learning Objectives
- to describe and measure the pressure of a gas.
At the macroscopic level, a complete physical clarification of a sample of a gas requires four quantities:
- temperature (expressed in kelvins),
- volume (expressed in liters),
- amount (expressed in moles), and
- pressure (in atmospheres).
Equally we demonstrated below, these variables are not contained (i.east., they cannot be arbitrarily be varied). If we know the values of any iii of these quantities, we can summate the fourth and thereby obtain a full physical description of the gas. Temperature, volume, and amount have been discussed in previous capacity. We at present discuss pressure level and its units of measurement.
Units of Pressure
Whatever object, whether it is your figurer, a person, or a sample of gas, exerts a force on any surface with which information technology comes in contact. The air in a balloon, for instance, exerts a force against the interior surface of the balloon, and a liquid injected into a mold exerts a force confronting the interior surface of the mold, just every bit a chair exerts a force against the floor because of its mass and the effects of gravity. If the air in a balloon is heated, the increased kinetic energy of the gas somewhen causes the balloon to flare-up because of the increased pressure (\(P\)) of the gas, the forcefulness (\(F\)) per unit area (\(A\)) of surface:
\[P=\dfrac{\rm Forcefulness}{\rm Area}=\dfrac{F}{A}\label{10.2.1}\]
Pressure level is dependent on both the force exerted and the size of the surface area to which the force is applied. We know from Equation \(\ref{10.2.1}\) that applying the same force to a smaller area produces a higher pressure. When we use a hose to launder a car, for example, we can increase the pressure of the h2o past reducing the size of the opening of the hose with a thumb.
The units of pressure are derived from the units used to measure strength and area. The SI unit for pressure, derived from the SI units for force (newtons) and area (square meters), is the newton per square meter (\(N/yard^2\)), which is chosen the Pascal (Pa), after the French mathematician Blaise Pascal (1623–1662):
\[\rm 1 \;Pa=1\;Due north/m^2 \label{ten.2.2}\]
Example \(\PageIndex{1}\)
Assuming a paperback book has a mass of 2.00 kg, a length of 27.0 cm, a width of 21.0 cm, and a thickness of 4.5 cm, what pressure level does it exert on a surface if information technology is
- lying flat?
- standing on edge in a bookcase?
Given: mass and dimensions of object
Asked for: pressure
Strategy:
- Calculate the strength exerted past the book and and then compute the expanse that is in contact with a surface.
- Substitute these two values into Equation \(\ref{10.2.i}\) to find the pressure level exerted on the surface in each orientation.
Solution:
The forcefulness exerted by the book does not depend on its orientation. Recall that the force exerted by an object is F = ma, where m is its mass and a is its dispatch. In Earth'southward gravitational field, the acceleration is due to gravity (ix.8067 m/due south2 at Earth'southward surface). In SI units, the force exerted past the book is therefore
\[F = ma = 2.00 \;\rm kg\times ix.8067 \dfrac{\rm thousand}{\rm due south^2} = nineteen.6 \dfrac{\rm kg·grand}{\rm s^2} = 19.6\;\rm North \nonumber\]
A Nosotros calculated the forcefulness as nineteen.half dozen N. When the book is lying apartment, the area is
\[A=\rm0.270 \;m\times0.210 \;grand= 0.0567 \;m^ii. \nonumber\]
B The pressure exerted by the text lying flat is thus
\[P=\dfrac{F}{A}=\dfrac{19.six\;\rm N}{0.0567\;\rm grand^2}=3.46\times10^2 \rm Pa \nonumber\]
A If the book is standing on its end, the strength remains the same, but the expanse decreases:
\[\rm A=\rm21.0 \;cm\times4.five \;cm = 0.210 \;thousand\times0.045 \;m = 9.5 \times ten^{−iii} \;\rm m^2 \nonumber\]
B The pressure exerted by the text lying flat is thus
\[P=\dfrac{xix.vi\;\rm N}{9.5\times10^{-3}\;\rm grand^2}=2.06\times10^three \;\rm Pa \nonumber\]
Practice \(\PageIndex{ane}\)
What pressure does a threescore.0 kg student exert on the floor
- when continuing flat-footed in the laboratory in a pair of tennis shoes (the surface surface area of the soles is approximately 180 cmii)?
- as she steps heel-starting time onto a dance flooring wearing high-heeled shoes (the area of the heel = i.0 cm2)?
- Answer a
-
three.27 × teniv Pa
- Answer b
-
five.ix × 106 Pa
Barometric Pressure level
Just every bit we exert pressure on a surface because of gravity, so does our atmosphere. We live at the bottom of an ocean of gases that becomes progressively less dense with increasing distance. Approximately 99% of the mass of the atmosphere lies within 30 km of Earth's surface (Figure \(\PageIndex{1}\)). Every point on World's surface experiences a net pressure called barometric pressure level . The pressure exerted past the atmosphere is considerable: a one thousandii column, measured from sea level to the superlative of the atmosphere, has a mass of most x,000 kg, which gives a pressure of about 101 kPa:

Barometric pressure tin can be measured using a barometer, a device invented in 1643 by ane of Galileo'due south students, Evangelista Torricelli (1608–1647). A barometer may be constructed from a long glass tube that is airtight at one cease. Information technology is filled with mercury and placed upside down in a dish of mercury without assuasive any air to enter the tube. Some of the mercury will run out of the tube, merely a relatively tall cavalcade remains within (Figure \(\PageIndex{two}\)). Why doesn't all the mercury run out? Gravity is certainly exerting a down strength on the mercury in the tube, but information technology is opposed by the pressure level of the atmosphere pushing downwardly on the surface of the mercury in the dish, which has the net effect of pushing the mercury up into the tube. Because there is no air above the mercury inside the tube in a properly filled barometer (it contains a vacuum), there is no pressure pushing down on the column. Thus the mercury runs out of the tube until the pressure exerted by the mercury column itself exactly balances the pressure of the atmosphere. The force per unit area exerted past the mercury column tin can be expressed as:
\[\begin{marshal} P&=\dfrac{F}{A} \\[4pt] &= \dfrac{mg}{A} \\[4pt] &= \dfrac{\rho V\cdot g}{A} \\[4pt] &= \dfrac{ \rho \cdot Ah\cdot k}{A} \\[4pt] &= \rho gh \end{align}\]
with
- \(g\) is the gravitational acceleration,
- \(m\) is the mass,
- \(\rho\) is the density,
- \(V\) is the book,
- \(A\) is the bottom area, and
- \(h\) is pinnacle of the mercury cavalcade.
Nether normal conditions conditions at sea level, the ii forces are counterbalanced when the meridian of the mercury cavalcade is approximately 760 mm higher up the level of the mercury in the dish, as shown in Figure \(\PageIndex{two}\). This value varies with meteorological conditions and altitude. In Denver, Colorado, for example, at an summit of nearly i mile, or 1609 m (5280 ft), the height of the mercury column is 630 mm rather than 760 mm.
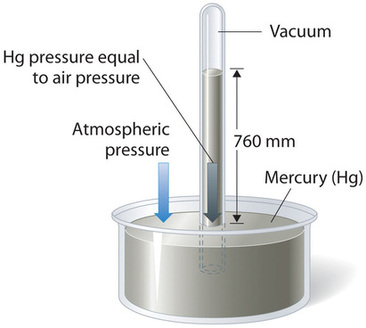
Mercury barometers have been used to mensurate barometric pressure level for so long that they have their ain unit for force per unit area: the millimeter of mercury (mmHg), often chosen the torr, after Torricelli. Standard barometric pressure level is the barometric pressure level required to back up a column of mercury exactly 760 mm alpine; this pressure is also referred to every bit 1 atmosphere (atm). These units are also related to the pascal:
\[\begin{align} \rm 1\; atm &= 760 \; mmHg \\[4pt] &= 760 \; torr \\[4pt] &= ane.01325 \times ten^5 \; Pa \\[4pt] &= 101.325 \; kPa\characterization{10.two.3} \cease{align}\]
Thus a pressure level of 1 atm equals 760 mmHg exactly.
We are and then accustomed to living under this pressure that we never notice it. Instead, what we notice are changes in the force per unit area, such as when our ears pop in fast elevators in skyscrapers or in airplanes during rapid changes in altitude. We brand utilize of barometric pressure in many ways. We tin can use a drinking harbinger because sucking on it removes air and thereby reduces the pressure inside the straw. The barometric pressure pushing down on the liquid in the drinking glass then forces the liquid up the straw.
Case \(\PageIndex{2}\): Barometric Pressure
One of the authors visited Rocky Mountain National Park several years ago. After departing from an aerodrome at sea level in the eastern United states, he arrived in Denver (altitude 5280 ft), rented a automobile, and collection to the summit of the highway outside Estes Park (height 14,000 ft). He noticed that even slight exertion was very hard at this altitude, where the barometric force per unit area is simply 454 mmHg. Convert this pressure level to
- atmospheres (atm).
- bar.
Given: pressure in millimeters of mercury
Asked for: pressure in atmospheres and bar
Strategy:
Use the conversion factors in Equation \(\ref{10.ii.3}\) to convert from millimeters of mercury to atmospheres and kilopascals.
Solution:
From Equation \(\ref{10.2.3}\), nosotros have 1 atm = 760 mmHg = 101.325 kPa. The pressure at xiv,000 ft in atm is thus
\[ \begin{align} P &=\rm 454 \;mmHg\times\dfrac{ane\;atm}{760\;mmHg} \\[4pt] &= 0.597\;atm \nonumber \cease{align} \nonumber\]
The force per unit area in bar is given by
\[ \brainstorm{marshal} P&=\rm 0.597\;atm\times\dfrac{ane.01325\;bar}{one\;atm}\\[4pt] &= 0.605\;bar \nonumber \terminate{align} \nonumber\]
Practise \(\PageIndex{2}\): Barometric Pressure
Mt. Everest, at 29,028 ft above sea level, is the world'due south tallest mountain. The normal barometric pressure at this altitude is about 0.308 atm. Convert this force per unit area to
- millimeters of mercury.
- bar.
- Answer a
-
234 mmHg;
- Answer b
-
0.312 bar
Manometers
Barometers measure barometric pressure, merely manometers measure the pressures of samples of gases contained in an apparatus. The key characteristic of a manometer is a U-shaped tube containing mercury (or occasionally another nonvolatile liquid). A closed-terminate manometer is shown schematically in part (a) in Figure \(\PageIndex{iii}\). When the bulb contains no gas (i.due east., when its interior is a near vacuum), the heights of the ii columns of mercury are the same because the infinite above the mercury on the left is a near vacuum (it contains but traces of mercury vapor). If a gas is released into the bulb on the correct, it will exert a pressure on the mercury in the right column, and the 2 columns of mercury will no longer exist the same tiptop. The deviation between the heights of the two columns is equal to the pressure level of the gas.
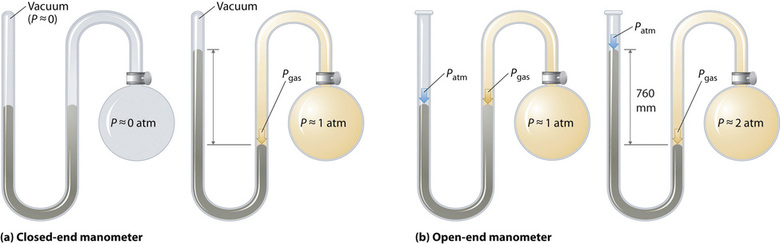
If the tube is open to the temper instead of airtight, equally in the open up-end manometer shown in part (b) in Figure \(\PageIndex{3}\), then the 2 columns of mercury take the aforementioned summit just if the gas in the bulb has a force per unit area equal to the barometric pressure. If the gas in the bulb has a higher pressure, the mercury in the open up tube will be forced up past the gas pushing down on the mercury in the other arm of the U-shaped tube. The force per unit area of the gas in the seedling is therefore the sum of the barometric pressure (measured with a barometer) and the deviation in the heights of the two columns. If the gas in the seedling has a pressure less than that of the atmosphere, and so the superlative of the mercury will be greater in the arm fastened to the bulb. In this case, the pressure of the gas in the seedling is the barometric pressure minus the difference in the heights of the two columns.
Example \(\PageIndex{iii}\)
Suppose you want to construct a airtight-cease manometer to measure gas pressures in the range 0.000–0.200 atm. Because of the toxicity of mercury, you decide to employ water rather than mercury. How tall a column of water practice you need? (The density of water is ane.00 g/cmiii; the density of mercury is 13.53 grand/cm3.)
Given: pressure range and densities of h2o and mercury
Asked for: column height
Strategy:
- Calculate the height of a column of mercury corresponding to 0.200 atm in millimeters of mercury. This is the height needed for a mercury-filled column.
- From the given densities, use a proportion to compute the height needed for a water-filled column.
Solution:
A In millimeters of mercury, a gas pressure of 0.200 atm is
\[P=\rm 0.200\;atm\times\dfrac{760\;mmHg}{i\;atm}=152\;mmHg\]
Using a mercury manometer, y'all would need a mercury column at least 152 mm loftier.
B Because water is less dense than mercury, y'all demand a taller column of h2o to achieve the same pressure equally a given column of mercury. The elevation needed for a water-filled column corresponding to a pressure level of 0.200 atm is proportional to the ratio of the density of mercury to the density of water
\[P=d_{\rm wat}gh_{\rm wat}=d_{\rm Hg}gh_{\rm Hg}\]
\[h_{\rm wat}=h_{\rm Hg}\times\dfrac{d_{\rm Hg}}{g_{\rm wat}}=\rm152\;mm\times\dfrac{13.53\;g/cm^3}{1.00\;g/cm^three}=2070\;mm\]
The respond makes sense: it takes a taller column of a less dense liquid to achieve the same force per unit area.
Exercise \(\PageIndex{iii}\)
Suppose you want to design a barometer to measure barometric pressure level in an environment that is always hotter than 30°C. To avert using mercury, y'all decide to utilise gallium, which melts at 29.76°C; the density of liquid gallium at 25°C is vi.114 g/cm3. How tall a cavalcade of gallium practice y'all need if P = 1.00 atm?
- Respond
-
1.68 1000
The reply to Example \(\PageIndex{iii}\) also tells us the maximum depth of a farmer's well if a simple suction pump volition be used to get the water out. The 1.00 atm corresponds to a column acme of
\[\begin{align} h_{\rm wat} &=h_{\rm Hg}\times\dfrac{d_{\rm Hg}}{g_{\rm wat}} \nonumber \\[4pt] &=\rm760\;mm\times\dfrac{thirteen.53\;yard/cm^3}{1.00\;g/cm^3} \nonumber \\[4pt] &= ane.03\times10^iv\;mm \nonumber \\[4pt] &= 10.iii\;m \nonumber \end{marshal} \nonumber\]
A suction pump is just a more sophisticated version of a harbinger: information technology creates a vacuum to a higher place a liquid and relies on barometric pressure to force the liquid up a tube. If i atm force per unit area corresponds to a x.3 m (33.8 ft) cavalcade of water, and so information technology is physically impossible for barometric pressure to raise the water in a well college than this. Until electric pumps were invented to button water mechanically from greater depths, this factor greatly limited where people could live because obtaining water from wells deeper than about 33 ft was difficult.
Summary
Pressure level is defined as the strength exerted per unit of measurement surface area; it can be measured using a barometer or manometer. Four quantities must be known for a consummate physical description of a sample of a gas: temperature, volume, amount, and pressure. Pressure is force per unit of measurement surface area of surface; the SI unit for force per unit area is the pascal (Pa), divers every bit 1 newton per square meter (Due north/m2). The pressure exerted by an object is proportional to the strength it exerts and inversely proportional to the area on which the strength is exerted. The pressure exerted by Earth's atmosphere, called barometric pressure, is about 101 kPa or 14.seven lb/in.ii at body of water level. barometric pressure level can be measured with a barometer, a closed, inverted tube filled with mercury. The tiptop of the mercury column is proportional to barometric pressure, which is often reported in units of millimeters of mercury (mmHg), also called torr. Standard barometric force per unit area, the force per unit area required to support a column of mercury 760 mm tall, is still some other unit of pressure: 1 atmosphere (atm). A manometer is an appliance used to measure out the pressure of a sample of a gas.
Source: https://chem.libretexts.org/Bookshelves/General_Chemistry/Map:_Chemistry_-_The_Molecular_Nature_of_Matter_and_Change_%28Silberberg%29/05:_Gases_and_the_Kinetic-Molecular_Theory/5.02:_Gas_Pressure_and_Its_Measurement
0 Response to "What four quantities are needed to accurately describe a gas?"
Post a Comment